Pythagorean Theorem For Stair Building
The Pythagorean Theorem:
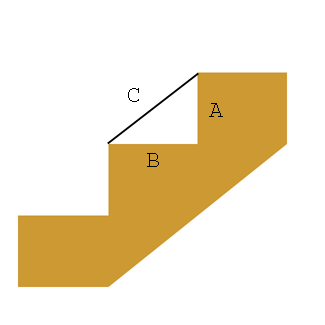
The sum of the areas of the two squares on the legs (A and B) equals the
area of the square on the hypotenuse (C). The theorem is as follows:
In any right triangles, the area of the square whose side is the
hypotenuse (the side opposite the right angle) is equal to the sum of
the areas of the squares whose sides are the two legs (the two sides
that meet at a right angle).
The square on the hypotenuse is equal to the sum of the squares of the
other two sides.
If we let C be the length of the hypotenuse and A and B, the lengths of
the other two sides, the theorem can be expressed as the equation.
A multiplied times A + B multiplied times B = C
multiplied times C
Example: The stair riser height of A is 7 inches and
the stair tread length is 10 inches.
7 x 7 = 49
10 x 10 = 100
Next Step 49 + 100 = 149
Next Step You will need to find the square root of 149.
On your calculator it will be a symbol that looks like this:
Next Step Enter 149 into the calculator and press the
square root symbol and the answer should be 12.20655
C would equal 12 and 1/4 inches. Now just multiply
12.2 times the number of steps or treads to get the length of the stair
stringer. This is helpful when figuring out your stringer lengths,
before purchasing them.
Using A Calculator To Figure Stair Risers